Great success in the development of the ocean model ICON-O
ICON is a joint development project between MPI-M and the German Meteorological Service (DWD). Through ICON, MPI-M uses new technologies and possibilities in high-performance computing. The ocean component of ICON, named ICON-O, is a state-of-the art ocean general circulation model on unstructured grids. It was developed independently at MPI-M and implements introduces a new computational methodology.
In detail: The first study addresses two parameterizations of oceanic processes. Parametrizations aim to model the impact of processes that can not explicitly be represented on the model grid due to a lack of resolution. The paper investigates the “isoneutral diffusion” and the “eddy parametrization” of Gent-McWilliams. They are milestones in ocean modelling and are applied by virtually every ocean general circulation model.
The actual computation of the parametrizations poses a subtle discretization problem, due to the nonlinearity of the parametrizations. Since the late 1990´s, only one solution to this problem was known. This discretization problem is considerably exacerbated on grids that are unstructured, such as ICON’s triangular grid. Now, after 20 years, Peter Korn´s study presents a new solution. This solution uses inherently unstructured grid methods. The paper shows through theoretical analysis and numerical experiments, ranging from idealized to global ocean simulations, that the new discretization preserves important physical properties of the two parametrizations.
In the second paper, Peter Korn and his colleague Leonidas Linardakis continue the analysis of the computational method of ICON-O. This is done by applying ICON-O’s discretization to the shallow water equations. The shallow water equations are a simplified set of equations of geophysical fluid dynamics that nevertheless contain some of the key aspects of the atmospheric or oceanic circulation. This has made shallow-water equations a popular numerical playground on which model properties, such as wave propagation and conservation properties of actual model codes, can be tested against theoretical and numerical benchmarks. The remarkable property of ICON-O is, that it preserves preserves both total energy and potential enstrophy in shallow-water discretization. Most other schemes preserve only one of them. The conservation of both quadratic invariants puts the numerical scheme in the same category as the famous Arakawa discretization from 1990.
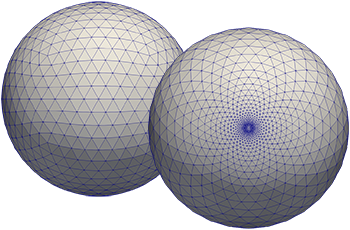
A second contribution of the paper is the proposal of a new triangular grid with a non-uniform resolution. The resolution increases from the equator towards the poles and creates a mesh that appears like a triangular version of a latitude-longitude grid. The ICON-O code runs on this grid without model changes. Through the use of two grids, the authors also demonstrate the robustness of numerics and the flexibility of the ICON-O code.
Original publications:
Korn, P.: A Structure Preserving Discretization of Ocean Parametrizations on Unstructured Grids. Ocean Modelling 132 (2018), 73-90. doi.org/10.1016/j.ocemod.2018.10.002
Korn, P. & L. Linardakis: A conservative discretization of the shallow-water equations on triangular grid. J. Comp. Phys. 375 (2018), 871-900. doi.org/10.1016/j.jcp.2018.09.002
Contact:
Dr. Peter Korn
Max Planck Institute for Meteorology
Phone: +49 40 41173 470
Email: peter.korn@mpimet.mpg.de
